RC Circuits

"A fact is a simple statement that everyone believes. It is innocent, unless found guilty. A hypothesis is a novel suggestion that no one wants to believe. It is guilty, until found effective"

Edward Teller

CHARGING

An example of a series RC circuit is shown at right. With the emf included in the circuit, applying the loop theorem we find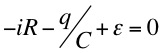

where q/C is the voltage drop across the capacitor and i is the current in the circuit.

Using the fact that i = dq/dt, we obtain

eqn2

This is a "simple" differential equation the solution of which can be written

or

The voltage across the capacitor, V_C = q/C and the voltage across the resistor, V_R = iR. Using the equations above we find that the dependence of these voltages on time is shown below

fig2

Note that the time on the horizontal axis is measured in units of τ = RC, the capacitative time constant.

After one time constant V_C has reached 63% (1 - e^-1) of its maximum value and V_R has 37% (1/e) of its final value.

divider

DISCHARGING

Now switch the emf out of the circuit and reapply the loop theorem

which gives

which has the solution

and

where Cε is the initial charge on the capacitor and ε/R is the initial voltage across the capacitor.

The time dependence of V_C and V_R (where V_R is proportional to the current in the capacitor) are shown at right.

Once again the time axis is measured in units of RC.

After one time constant V_C has decreased to 37% (1/e) of its initial value and |V_R| has decreased to 37% (1/e) of its initial value.

Q: What did one quantum physicist say when he wanted to fight another quantum physicist?
A: Let me atom.

Dr. C. L. Davis
Physics Department
University of Louisville
email: c.l.davis@louisville.edu